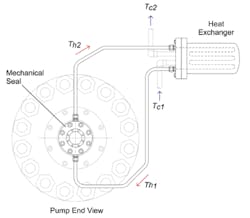
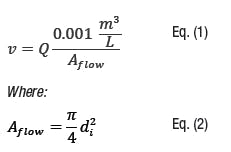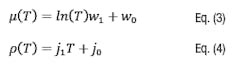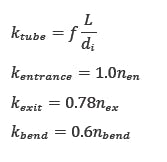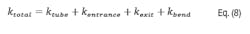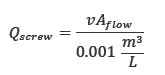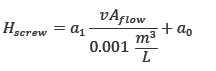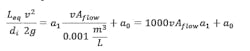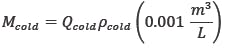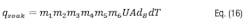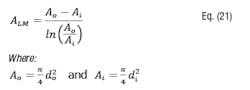Establishing a mechanical seal baseline

Figure 1. Plan 23 systems installed on a high-pressure multistage pump

Oil refineries began using the precursor to API Plan 23 to seal light hydrocarbons that had to be cooled to prevent flashing and short mechanical seal life. Circulating devices were added to the seal design to circulate the process fluid through a shell and tube heat exchanger in a nearly closed loop. Decades later, API Plan 23 has proven to be the most effective way of sealing hot fluids in centrifugal pumps from a reliability, safety and economic perspective. These systems, extended from refining to power generation, are used widely today in water applications above 80 C, such as boiler feedwater services.

Detailed design calculations are not required in most cases where the application is a typical Plan 23, the mechanical seal contains a well designed circulating device, best practices are used for the layout and piping of the loop, and a well designed heat exchanger with known performance characteristics is used. But in some cases where one or more of these parameters is unusual, the application is hazardous, plant critical, or a due diligence study is advisable, then predictive calculations can be performed.

These calculations can be used as a baseline to determine if the mechanical seal is leaking, the Plan 23 tubing has been clogged or fouled, or the cooling water supply is insufficient. In each case, one will see a rise in the Plan 23 loop temperatures over time. If the performance parameters are measured in the field, this algorithm can be altered empirically for further comparison. Altering the overall heat-transfer coefficient is usually the best way to do this.

The method introduced here is iterative. This is due to:

The use of the log mean temperature difference (LMTD) used in determining heat exchange rate
The determination of the mass flowrate of the recirculated process fluid as a function of friction losses
Some parameters are nonlinear functions, such as:

Absolute viscosity as a function of temperature (exponential curve fit)
Friction losses (empirical)
Head-versus-flow function of the circulating device (curve fit)

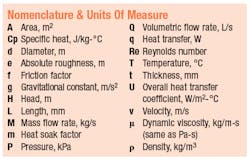
In order to achieve accuracy, the following input parameters are required:

The operating temperature of the process fluid,
Liquid density and viscosity as functions of temperature,
Dimensionless factors m₁ through m₆, as defined by Buck and Chen (2010),
The head-versus-flow curve for the circulating device in the mechanical seal,
Several dimensions of the seal cooler and interconnecting piping,
The flowrate and temperature of the cooling fluid entering the seal cooler,
Mechanical seal generated heat.
At the end of this article, some rule-of-thumb values for quick "back of the envelope" calculations will be included. These are valuable when one does not have a detailed description of the mechanical seal, the seal cooler or pump construction.

The physical system of a plan 23

The calculations in this method are based on a shell and tube cooler wherein the process fluid flows through the tube side, and the cooling liquid flows through the shell side (Figure 1). (One can easily modify these calculations for other cooler configurations.)

Plan 23 loop flowrate

We will need a Plan 23 mass flowrate, M_hot, in order to calculate the rate of heat transfer, q_cooler. This flowrate is generated by the circulating device in the mechanical seal. There are two basic types of circulating devices used for mechanical seals: radial circulating rings and positive-displacement pumping screws. Each has a characteristic curve as shown below. A radial circulating ring curve can be approximated by a second order curve as shown. A pumping screw curve is linear, as can be expected from a positive-displacement device.

The flow velocity for a flow Q:

The Reynolds number is calculated to determine if the flowrate is laminar or turbulent. The Reynolds number equation contains the viscosity term, μ, and the density term, ρ. The viscosity of nearly all liquids can be characterized as an exponential function of temperature Density of a liquid is a nearly linear function of temperature. The density change across the cooler and the mechanical seal can be 1-5 percent (particularly within the iteration steps), so this function must be included on the hot side of the Plan 23 loop. So, the two terms are modeled as:

For clean water, these terms are:
W₀ = −4.7e − 4, w₁ = 0.0024,j₀ = 1009, j₁ = −0.441

(These constants must be consistent with the units specified above.)

The average temperature of the liquid in the Plan 23 loop, T, will be an iterative value as shown later.

Reynolds numbers can range from 1,000 to 6,000 when the Plan 23 flow is on the shell side of the cooler, so the laminar or turbulent check is important. The friction factor f is calculated as follows. A slight discontinuity will exist at Re = 2,100. Friction factor relationships that can model the entire laminar-transitionturbulent range exist but are very difficult to evaluate, and the increase in accuracy and continuity do not justify their use.¹

The dimensionless friction factors, k, can be determined.

Where n is the number of entrances or exits in the system loop. There will be two of each: in and out of the seal; in and out of the cooler. One can add k factors for other resistive causes, such as tees, elbows, valves, but since these devices are not recommended, they are ignored. The overall friction factor k_total is the sum of all k’s.

The equivalent length of the piping system is

And now the head loss H_f can be calculated for any flow velocity v.

A pumping screw device in a mechanical seal will have a linear head versus flow relationship of the form:

where a₁ and a₀ are constants specific to the screw dimensions, fluid and speed. The flow generated by the pumping screw Q_screw will enter the interconnecting Plan 23 tubing, which has a flow area A_flow, calculated above.

Substituting this into the pumping screw relation

The flow velocity through the Plan 23 tubing will be that at which H_f is equal to H_screw. Setting these two relations equal to one another gives

This can be arranged as a quadratic equation with v as the variable and the other terms constants:

Which will produce a positive and negative value of v, and we select the positive one. Finally, the mass flowrate, M, can be determined.

The mass flowrate of the cooling fluid is calculated. Since the flowrate of the cooling fluid is assumed to be constant and relatively high (0.5 to 1.25 L/s), and the temperature rise through the cooler should not be greater than about 10 Δ°C, the change in density will be less than 1 percent and can be considered constant.

The heat transfer system

There are two main sources of heat flowing into the Plan 23 process liquid—heat soak, q_soak, and seal generated heat, q_seal. There is one significant source of heat removal—the seal cooler, q_cooler. If these sources are at steady state then the heat transfer balance equation is:

q_soak + q_seal – q_cooler = 0

From Buck and Chen (2010)², heat soak may be calculated as follows:

Where m₁ through m₆ are the dimensionless factors used to correct the equation recommended by API 682, which recommends a singular UA value of:

Where T_process is the process liquid bulk temperature in the pump casing and T_chm is the average bulk temperature in the seal chamber. If the pump has a cooling jacket in front of the seal, it is acceptable to use q_soak = 0.

The seal generated heat, q_seal, can be calculated using the method recommended by API 682 or obtained from the mechanical seal manufacturer. The heat transfer occurring across the heat exchanger can be calculated three ways, all of which are required in this method.

In these three equations, the subscripts hot and cold refer to the side of the heat exchanger containing the process fluid and the side containing the cooling liquid, respectively. The dT values are calculated as follows:

The log mean area is used because the U value used for the heat exchanger is an overall heat transfer coefficient defined neither by the OD nor the ID of the tubes:

The overall heat transfer coefficient, U_cooler, can be calculated using several different empirical formulas, but one can obtain good accuracy by using curve fit functions or singular values obtained through testing. (520 W/m2-°C is a reliable value to use for modern seal coolers supplied by seal manufacturers.)

Now we have the system of equations that govern the Plan 23 flow and heat transfer relationships. Unfortunately we cannot solve for the unknown temperatures T_h1, T_h2 and T_c2 because of the nonlinear log mean temperature equation for d_TLM. We cannot solve directly for the mass flowrate through the system because the viscosity and density terms in the Reynolds equation are functions of the unknown temperatures and the nonlinear expression for the friction factor f_turbulent used if the flow is in the turbulent regime. But several methods of solving a system of nonlinear equations exist. Most methods use a convergence criterion, which defines when a solution has been found. For our problem, one could keep guessing at T_h1 until Eq. (15) has been satisfied to within 0.5 percent or so. Using the Solver function in Microsoft Excel is one of the easiest ways to solve this system of equations (see "Solution Example" sidebar).

Quick calculations

The following values and calculations can be used for simple hot process water analysis where many of the detailed variables above are unknown. This method is useful for troubleshooting applications in the field or sizing heat exchangers or cooling water requirements for budgetary proposals:

The actual loop temperatures, T_h1 and T_h2, will be functions of seal generated heat and heat soak (neither of which are measurable in the field) but should each be between 30 C and 50 C, and the dT_hot should be between 2 and 4 Δ°C.

The results of the quick calculations are:

References

See McKeon, B.J., C.J. Swanson, M.V. Zaragola, R.J. Donnelley, and A.J. Smits. “Friction Factors for Smooth Pipe Flow.” J. Fluid Mech. 511 (2004): 41-44.
Buck, Gordon S., and Tsu Yen Chen. An Improved Heat Soak Calculation for Mechanical Seals. Proc. of Proceedings of the 26th International Pump Users Symposium, Houston, TX, 2010.

John Merrill is the U.S. principal engineer for EagleBurgmann, a manufacturer of mechanical seals and sealing solutions. Mr. Merrill has been designing, installing, troubleshooting, and testing mechanical face seals and seal support systems since 1991. His market sector experience includes upstream and downstream oil and gas, mining, chemical production, biopharmaceuticals, fossil and nuclear power generation. Mr. Merrill currently serves as chairman of the Hydraulic Institute’s Seals Committee and participates in the Reliability Committee. He can be reached at [email protected] or 704 525-9672.